Level Up Your Math Game: Practice Algebra Problems for the SAT
You’ve set your sights on college; the SAT is a necessary hurdle. The Math section can be particularly tough among the challenges you’ll face.
This guide tackles a crucial component of the SAT Algebra Questions. We’ll get into its importance and equip you to conquer the different algebra problems you’ll encounter.
However, what importance does algebra hold in the SAT? Let’s find out!
Why Algebra Matters on the SAT
Algebra is crucial for the SAT Math section, as it constitutes 35% of the questions. This means 13–14 questions will focus on algebraic concepts. The section tests your ability to think critically and solve problems involving:
- Linear Equations and Inequalities: Analyzing and solving equations and inequalities in one variable.
- Systems of Equations and Inequalities: Solving and interpreting systems of equations and inequalities.
Mastering these skills is essential, as they form the foundation for a significant portion of the SAT Math section. Understanding and practicing these concepts will greatly enhance your test performance and overall score.
What Algebra Skills will the SAT Math Section Test?
The SAT algebra problems are a way of testing your grasp of different concepts.
Here are the skills on which you will be tested for your SAT algebra questions skills:
Key Areas
- Linear equations in one variable
- Linear equations in two variables
- Linear functions
- Systems of two linear equations in two variables
- Linear inequalities in one or two variables
Remember, this is just a glimpse into the SAT algebra questions you might encounter. The key is to be comfortable with various concepts and have a strategic approach to solving them.
Now, moving on to the next section, we’ll familiarize you with the different questions asked in algebra!
Also Read: Crucial Tips and Tricks to Effectively Utilize Math Reference Sheet
Equation Solving and Absolute Values
Absolute values might seem intimidating at first glance, but they’re a straightforward concept that can be mastered with practice.
Today, we’ll unveil the basics of absolute values in equations, equipping you to solve them confidently.
Understanding the Concept
Imagine a number line stretching infinitely in both directions, positive and negative. The absolute value of any number, represented by ||, simply tells you its distance from zero on that line.
So, whether a number is positive or negative, its absolute value is always non-negative.
For instance, the absolute value of 5 is 5, and the absolute value of -7 is also 7.
Now, absolute values become particularly interesting when they show up in equations. They introduce a twist because an absolute value can represent two solutions – one positive and one negative – with the same distance from zero.
Step-by-step process to solve equations involving absolute values
Let’s break down the process of solving equations involving absolute values:
- Isolate the Absolute Value: Maneuver the equation so the absolute value term sits alone on one side. This is like clearing away any clutter around the absolute value to get a good look at what’s inside.
- Split into Two Equations: Since the absolute value can represent either a positive or negative solution, we acknowledge this by creating two separate equations.
- The first equation sets the expression inside the absolute value brackets equal to a positive value (without the absolute value symbol).
- The second equation sets the expression inside the brackets equal to its negative counterpart.
- Solve Each Equation Independently: Treat these newfound equations like any other linear equation. Solve for each variable, remembering to pay attention to any extraneous solutions that might pop up.
- Combine the Solutions (Carefully): Once you have the solutions from both equations, you’ve conquered most of the challenge! However, remember that absolute values only care about the distance from zero, not the direction (positive or negative). So, your final answer will include both the positive and negative solutions you found, as long as they make sense in the context of the original equation.
Putting Theory into Practice: Let’s Solve an Example!
Here’s a problem to test your newfound skills:
|x + 3| = 5
Following the steps above:
- Isolate the absolute value: We want to get x by itself, so let’s subtract 3 from both sides: |x| = 2
- Split into two equations:
- Equation 1: x = 2 (positive solution)
- Equation 2: x = -2 (negative solution)
- Solve each equation: This is simple addition/subtraction. We get x = 2 and x = -2.
- Combine solutions: The absolute value could represent either distance, so our final answer is x = 2 or x = -2.
Remember to Check! Always plug your solutions back into the original equation to ensure they hold true. In this case, both 2 and -2 work perfectly.
By understanding absolute values and following these steps, you’ll be well on your way to solving even the trickiest equations involving them!
Also Read: Why Everyone Got This SAT Math Question Wrong
Linear Equations and Inequalities
Linear equations and inequalities – these might sound like fancy terms, but they’re the building blocks of many SAT Math problems.
Let’s break them down, understand their importance, and equip you with the skills to tackle them effectively.
Understanding Linear Equations
Imagine a balanced scale. On one side, you have a variable (represented by letters like x or y), and on the other, you have constants (numbers that don’t change).
A linear equation is like that scale – an equal sign sits in the middle, ensuring that both sides “balance out” mathematically. These equations are crucial on the SAT because they test your ability to represent relationships between variables and solve for unknown values.
For instance, the equation 2x + 5 = 11 describes a relationship between x and a constant value (11). By solving this equation, you’d find the value of x that makes the scale balance (x = 3).
LearnQ.ai is Powered by VEGA AI—Is your Institute Next?
Give students a Duolingo-style test-prep platform with Shopify-level customization for tutors and institutes.
Understanding Inequalities
Linear inequalities take things a step further.
Instead of a strict equal sign, you’ll encounter symbols like < (less than), > (greater than), ≤ (less than or equal to), and ≥ (greater than or equal to). These inequalities represent a range of possible solutions, like saying a number needs to be “bigger than 5” or “less than or equal to 10.”
Understanding inequalities is key because they model real-life scenarios with restrictions.
Imagine you need to score at least 600 on the Math section (greater than or equal to 600). An inequality can help you determine the range of scores you need to achieve your goal.
Systematic Approach to Solving Linear Inequalities
Now that you grasp the concepts let’s tackle solving linear inequalities:
- Isolate the Variable: Just like with linear equations, your aim is to get the variable by itself. Use basic arithmetic operations (addition, subtraction, multiplication, and division) on both sides of the inequality, but remember to flip the inequality sign whenever you multiply or divide by a negative number (to maintain the balance).
- Identify the Solution Set: Based on the inequality symbol, you’ll end up with a range of values for the variable.
For example, x > 5 means your solution includes all numbers greater than 5 (think of an arrow pointing to the right on the number line).
Sample problem with explanation and solution strategies
Let’s test your newfound skills with this problem:
3x – 2 ≤ 10
- Isolate x: Add 2 to both sides, then divide both sides by 3. You’ll get x ≤ 4.
- Identify the solution set: Since we have ≤, the solution includes all values less than or equal to 4. Imagine shading everything on the number line to the left of 4, including 4 itself.
By following these steps and practicing with various inequalities, you’ll be well-equipped to tackle them on the SAT. Remember, the key is understanding the concept of balancing and manipulating the equation to isolate the variable within the designated range.
Temperature Conversion Problems
Math isn’t just about abstract equations – it has real-world applications!
Today, we’ll explore how algebra empowers you to tackle a practical challenge: temperature conversion.
Whether following a recipe or planning a trip abroad, understanding how to convert between Celsius (°C) and Fahrenheit (°F) is a valuable skill.
Procedure for Converting Celsius to Fahrenheit
Remember that algebra is the language of variables and equations.
In temperature conversion, we use variables to represent the unknown temperatures in Celsius (let’s call it “C”) and Fahrenheit (“F”). The conversion formula itself becomes an equation that relates these variables.
Here’s the formula for converting Celsius to Fahrenheit:
F = (C x 9/5) + 32
This equation tells us that to find the Fahrenheit value (F), we must perform a series of operations on the Celsius value (C). It involves multiplying C by 9/5 (which essentially converts it to a corresponding Fahrenheit scale) and then adding 32 to account for the difference between the freezing points of the two scales.
Example conversion problem with a comprehensive explanation
Imagine you’re baking a delicious cake, and the recipe calls for 180°C. But your oven only displays temperature in Fahrenheit.
We can use algebra to solve this:
- Plug in the Celsius value: We know C = 180. Substitute this value into the formula: F = ((180) x 9/5) + 32
- Perform the calculations: Following the order of operations, we get F = (324/5) + 32. Simplifying, we get F = 64.8 + 32.
- The answer: The cake needs to be baked at 348°F (rounded to the nearest whole number).
This example demonstrates how algebra empowers you to convert temperatures with ease. By understanding the formula and plugging in the values, you can conquer any conversion challenge!
Also Read: Pratice Digital SAT Math: Geometry Questions and Answer Worksheet
Age-related Algebra Problems
Algebra might seem abstract at times, but it can actually be quite relatable!
Today, we’ll get into age-related problems, which is a fun way to practice setting up and solving equations.
These problems often involve figuring out someone’s age based on given clues, making them practical and engaging.
Technique to find relationships between ages using equations
Age-related problems typically present you with a scenario involving two or more people’s ages. The key lies in translating the information into an algebraic equation.
Here’s the approach:
- Identify the Variables: Assign a variable (usually a letter like x or y) to represent the unknown age you’re trying to solve for. For example, if you need to find out Lisa’s age, you can represent it as “L.”
- Translate the Clues: The problem might say something like, “John is 5 years older than Lisa.” This translates into an equation: J = L + 5 (where J represents John’s age).
By translating the relationships between ages into equations, you create a system that allows you to solve for the unknown age.
Detailed Explanation of an Example Problem
Let’s tackle a sample problem to solidify your understanding:
Anna is currently twice as old as Ben. In five years, Anna will be 25 years old. How old is Ben now?
- Identify the Variables: Let M be Anna’s current age and B be Ben’s current age.
- Translate the Clues:
- Anna is twice as old as Ben: M = 2B (since twice something is the same as multiplying by 2)
- In five years, Anna will be 25: M + 5 = 25 (this translates to “Anna’s current age + 5 years = 25”)
Now, we have two independent equations, but our goal is to find B (Ben’s age).
Solving the Question Further:
The trick here is to use the information about Anna (M) to solve for Ben (B). Since we already have an equation for M in terms of B (M = 2B), let’s substitute this into the second equation:
(2B) + 5 = 25 (we replace M with its equivalent expression)
Now, we can solve for B:
- Simplify the equation: 2B + 5 = 25
- Subtract 5 from both sides: 2B = 20
- Divide both sides by 2: B = 10
Therefore, Ben is currently 10 years old.
By setting up equations and manipulating them strategically, you can unlock the age mysteries presented in these problems. So, the next time you encounter an age-related algebra problem, remember this approach and solve it!
Distance, Rate, and Time Problems
The world around us is full of motion, and algebra provides the tools to understand and quantify it.
Today, we’ll explore distance, rate, and time (DRT) problems – a fundamental concept in algebra with real-world applications.
Understanding the Formulae Related to Distance, Rate, and Time
Imagine you’re on a road trip. The distance you travel (D), your speed (rate, R), and the time it takes (T) are all interconnected. Luckily, we have a magical formula that ties them together:
D = R x T
This equation essentially says that the distance traveled (D) is equal to the rate (speed, R) multiplied by the time taken (T).
You can calculate any of these three variables if you know the other two.
Also Read: SAT Maths Tips and Tricks to Boost Your Score
Calculating Time or Rate by Applying Algebraic Equations
The beauty of algebra lies in its ability to isolate variables. In DRT problems, you might be given two values and need to solve for the third.
Here’s how to tackle them:
- Identify the Given Values: Look for the information provided about distance, rate, or time in the problem. Let’s say you know the distance (D) and the rate (R) but need to find the time (T).
- Rearrange the Formula: Remember, algebra is all about manipulating equations. To solve for T, we need to isolate it. Divide both sides of the formula (D = R x T) by R. This gives you T = D / R.
Now you have a new equation where T is by itself, ready to be solved for using the given values of D and R.
Solving a Practical Problem with Algebraic Principles
Let’s test your newfound skills with a practical scenario:
You’re cycling to a friend’s house 15 kilometers (km) away. You can maintain a steady speed of 5 km/hour (your rate). How long will it take you to get there?
- Identify the Given Values: Distance (D) = 15 km, Rate (R) = 5 km/hour (remember, speed is a type of rate)
- Solve for Time (T): We need to find T. Apply the formula we derived earlier: T = D / R. Plugging in the values, we get T = 15 km / 5 km/hour.
- Calculate the Time: Divide the distance by the rate. T = 3 hours.
So, it will take you 3 hours to cycle to your friend’s house.
By understanding the DRT formula and applying algebraic manipulation, you can solve various real-life problems involving distance, rate, and time.
Remember, the key is to identify the given values, rearrange the formula as needed, and perform the calculations to find the missing variable.
So, the next time you’re planning a trip or analyzing movement, put your DRT algebra skills to the test!
Also Read: Essential SAT Math Notes, Guides, and Facts
Systems of Equations
The SAT throws various curveballs, and systems of equations are no exception.
Here, you’re presented with two or more equations and tasked with finding the values that satisfy all of them simultaneously.
Imagine yourself as a detective – you need to gather clues from each equation to solve the case!
We’ll explore two powerful methods to tackle these systems and equip you for the SAT: substitution and elimination.
Methods to Solve Systems of Equations
Systems of equations are more than just a mathematical concept. They model real-world scenarios where multiple variables are interdependent.
For instance, you might encounter a system on the SAT that describes a mixture of chemicals or the combined cost of different items on sale.
There are two main approaches to solving systems of equations: substitution and elimination. Both have their advantages depending on the specific problem.
Here’s a breakdown:
- Substitution: This method involves solving one equation for a variable in terms of the other variable. Then, you “substitute” this expression into the second equation, replacing that variable with its equivalent form. Now, you have a single equation with one variable, which you can solve for. Once you have that variable’s value, you can plug it back into either original equation to solve for the remaining variable.
- Elimination: This method focuses on manipulating the equations to get one of the variables to “cancel out” when you add or subtract the equations. Here’s the trick: you want to modify the coefficients (the numbers multiplying the variables) in one equation so they become negative inverses of the coefficients in the other equation for that same variable. When you add or subtract the equations with these strategically modified coefficients, the targeted variable cancels out, leaving you with a single equation in terms of the other variable. Solve for that variable, and then substitute its value back into either original equation to find the first variable.
Example with Detailed Solution Process
Imagine you’re working at a bakery and need to mix two types of flour – whole wheat (w) and all-purpose (a).
Here’s a system you encounter:
2w + a = 10 (Equation 1) w + 3a = 15 (Equation 2)
We can solve this system using either substitution or elimination. Let’s try substitution:
- Solve Equation 1 for w: w = 10 – a (we isolate w by subtracting a from both sides).
- Substitute w in Equation 2: Replace w with its equivalent expression from Equation 1: (10 – a) + 3a = 15.
- Solve for a: Simplify the equation: 2a + 10 = 15. Subtract 10 from both sides and solve for a: 2a = 5, so a = 2.5.
- Solve for w: Now that you know a = 2.5, plug it back into either original equation. We’ll use Equation 1: 2w + 2.5 = 10. Solve for w: 2w = 7.5, so w = 3.75.
Therefore, you need 3.75 cups of whole wheat flour and 2.5 cups of all-purpose flour for your perfect recipe.
By understanding both substitution and elimination, you’ll be well-equipped to tackle various systems of equations you might encounter on the SAT.
Remember to choose the method that seems more efficient based on the specific problem and practice regularly to develop your problem-solving skills.
Comparison of Trajectories
Imagine you’re tracking the paths of two rockets or analyzing the growth patterns of two companies. In these scenarios, you’re dealing with trajectories – the paths or changes things take over time.
Algebra acts as a powerful tool to analyze and compare these trajectories, allowing you to understand their similarities and differences.
Analyzing Trajectories Using Algebra for Comparison
Trajectories are often represented by equations that express a variable (usually time, t) in relation to another variable (representing the changing quantity, like position, x, or growth rate, y).
These equations encode the “rules” governing how the quantity changes over time.
For instance, the equation x = 2t + 5 might represent the position (x) of a moving object at any given time (t). In this case, the object starts at position 5 and moves 2 units further every unit of time.
Finding Intercept Points of Trajectories with Algebra
When comparing trajectories, pinpointing where they intersect becomes crucial. These “intercept points” represent the times or values at which the two trajectories have the same outcome.
Finding them involves solving a system of equations, where each equation represents one trajectory.
Here’s the approach:
- Set Up the System: Write down the equations for both trajectories, ensuring each equation has the same variable representing time (t).
- Solve the System: Employ methods like substitution or elimination (covered earlier in the blog) to solve for the value of t that satisfies both equations simultaneously. This t-value represents the time at which the trajectories intersect.
Enhance your Digital SAT study routine with AI-driven insights and personalized practice tests.
Walkthrough of a Comparative Example with a Solution
Let’s say you’re comparing the temperatures of two different food items as they cool down.
Here are the equations representing their temperature changes:
- Food A: T_a = -2t + 100 (T_a represents the temperature of Food A at time t, and the equation shows it cools down at a rate of 2 degrees per unit of time, starting at 100 degrees)
- Food B: T_b = -3t + 120 (T_b represents the temperature of Food B, cooling down at 3 degrees per unit of time and starting at 120 degrees)
We want to find the time at which both foods have the same temperature.
- Set Up the System: We already have the equations for both trajectories.
- Solve the System: Since the variables (t) are already isolated, we can set the temperature expressions equal to each other: -2t + 100 = -3t + 120.
- Solve for t: Add 2t to both sides and subtract 100 from both sides: t = 20.
Therefore, both Food A and Food B will have the same temperature after 20 units of time.
By setting up equations and using algebraic techniques, you can analyze and compare trajectories in various scenarios.
This empowers you to understand how things change over time relative to one another, a valuable skill in many fields.
Remember, the key is translating the problem into a system of equations and solving for the desired information, like the intercept points in our example.
Also Read: An Overview of SAT Maths Formulas, Questions, and Strategies
Predicting and Solving Radical Equations
Radical equations, where variables hide under radical signs, can seem intimidating at first. But fear not! With a good understanding of their nature and a strategic approach, you can predict solutions and solve them like a pro.
What are Radical Equations?
Imagine an equation with a variable lurking under a radical symbol (like square root, cube root, etc.). That’s a radical equation! These equations challenge you to find the values of the variable that make the equation true, considering the properties of radicals.
Here’s the catch: sometimes, solving a radical equation can lead to extraneous solutions – values that satisfy the equation after you solve it but don’t actually make sense in the context of the original equation.
Strategies to Predict and Solve Radical Equations Accurately
Before diving headfirst into solving, a little prediction can go a long way:
Consider the Restrictions: Radicands (the expressions under the radical) cannot be negative for square roots and other even-powered radicals. This restriction helps eliminate nonsensical solutions from the start.
Isolate the Radical: Maneuver the equation to get the radical term by itself on one side. This prepares you to tackle it head-on.
Practice Questions with a Thorough Explanation of the Solving Process
Now, let’s put these tips into action with a practice problem:
Solve: √(x + 5) = 3
Prediction: Since the square root is even-powered, the radicand (x + 5) cannot be negative. So, x + 5 must be greater than or equal to zero (x ≥ -5). This eliminates any solutions that would make x + 5 negative.
Isolate the Radical: Square both sides of the equation to get rid of the radical symbol. Remember, squaring a radical is like “undoing” it, as long as the radicand is non-negative (which we predicted earlier). This gives us: x + 5 = 3^2 (or x + 5 = 9).
Solve the Linear Equation: Subtract 5 from both sides, and you’re left with x = 4.
Check for Extraneous Solutions: Plug x = 4 back into the original equation (√(4 + 5) = 3). This checks out, so 4 is a valid solution. However, remember our prediction – x ≥ -5. Let’s check another value within this range, say x = -1. Plugging it in (√(-1 + 5) = 3) results in an undefined value (square root of a negative number). This confirms that x = -1 is an extraneous solution and should be discarded.
Therefore, the only valid solution is x = 4.
Remember: Always check for extraneous solutions by plugging your answer back into the original equation, considering the restrictions on the radicand.
By understanding the properties of radicals, employing prediction strategies, and following a step-by-step approach, you can conquer even the trickiest radical equations!
Algebra in Cost Splitting Scenarios
Ah, the age-old question: how do we split the bill fairly? Whether it’s a pizza with friends, a weekend getaway, or shared groceries, algebra comes to the rescue!
Today, we’ll explore how to apply algebraic equations to solve cost-splitting scenarios with ease.
Application of Algebra in Calculating Shared Costs
Imagine you and your friends share a pizza that costs $15. You want to split the bill equally (fair and square!). Algebra allows you to represent the unknown amount each person owes with a variable. Let’s call it “x.” Now, the total cost translates into an equation:
Total Cost = Number of People × Cost per Person
In this case:
15 = x × (number of friends)
By solving this equation for “x,” you can find out how much each person owes.

An illustrative Example of Cost Splitting in a Real Scenario
Let’s say you and two friends are planning a weekend trip. You need to figure out how to split the following costs:
- Accommodation: $200 (total cost)
- Food: Let y represent the total cost of food (since it’s unknown)
- Gas: You’ll split gas money equally, but you don’t know the total cost yet. Let’s call it “z.”
Here’s how algebra helps:
- Accommodation: This cost is fixed, so each person owes a share of $200 / (number of friends).
- Food: You’ll need to determine the total food cost (y) and then divide it by the number of friends.
- Gas: Similar to food, you’ll need to find the total gas cost (z) and then split it equally.
The key is to identify the unknown variables and write equations to represent the total cost for each expense category.
Problem-Solving Strategy with a Worked-Out Example
Imagine you and your two friends (a total of 3 people) spend $80 on food (y) and $60 on gas (z).
Here’s how to find out how much each person owes:
- Accommodation: Each person owes $200 / 3 people = $66.67.
- Food: You now know the total food cost (y = $80). Split it equally: $80 / 3 people = $26.67 per person.
- Gas: The total gas cost is z = $60. Split it equally: $60 / 3 people = $20 per person.
Now comes the grand finale!
Total Cost per Person: Add up each person’s share for accommodation, food, and gas: $66.67 + $26.67 + $20 = $113.34.
Therefore, each person owes approximately $113.34 for the entire trip.
This is just one example, but the same approach can be applied to any cost-splitting scenario. By representing unknowns with variables and setting up equations, you can solve for each person’s fair share with confidence!
Identifying Non-Perpendicular Lines
Let’s shift our focus to– non-perpendicular lines. We’ll learn how to identify them using equations and ditch the confusion.
Concept of Perpendicular Lines in Algebra and Their Characteristics
Remember perpendicular lines? These are the picture-perfect right-angle buddies that intersect at exactly 90 degrees. In the land of equations, they have a special handshake:
- Slopes are negative reciprocals: The slope (m) of one line is the negative reciprocal of the slope of the other. Imagine a fraction flipped and multiplied by -1 – that’s the relationship!
For instance, a line with a slope of 2 would be perpendicular to a line with a slope of -1/2.
Techniques to Identify Non-Perpendicular Lines through Equations
Now, how do we identify lines that aren’t part of the perpendicular posse? Here are a couple of techniques:
- Slope Check: Look at the slopes of the two lines you’re comparing. If the slopes are not negative reciprocals of each other, the lines are not perpendicular. This is the quickest method, but it only works if you have the slope in explicit form (y = mx + b).
- Examine the Product of Slopes: Multiply the slopes of the two lines. If the product is equal to -1, the lines are perpendicular. If the product is not -1, the lines are not perpendicular. This method works even if you don’t have the slopes in explicit form, but you need to solve for the slopes first.
Problem Example with an Explanation on Identifying the Correct Line
Let’s say you have two lines:
- Line 1: y = 2x + 5 (slope = 2)
- Line 2: 3x – y = 1 (we need to re-arrange this equation to solve for the slope)
- Slope Check (Optional): Since the slope of Line 1 (2) is not the negative reciprocal of the slope of Line 2 (which we haven’t calculated yet), we know they’re not perpendicular right away.
- Calculate the Slope of Line 2: Move the x term to the other side and divide by -1 to get y = 3x – 1. Now, the slope is the coefficient of x (3).
- Product of Slopes: Multiply the slopes (2 x 3). The product is 6, not -1. Therefore, the lines are not perpendicular.
By using these techniques, you can confidently identify non-perpendicular lines in various scenarios.
Remember, the key is to understand the relationship between slopes and perpendicular lines and then choose the appropriate method to analyze the equations.
LearnQ.ai is powered by VEGA AI—Is your institute next?
Offer students a Duolingo-style test-prep platform with Shopify-level customization for tutors and institutes.





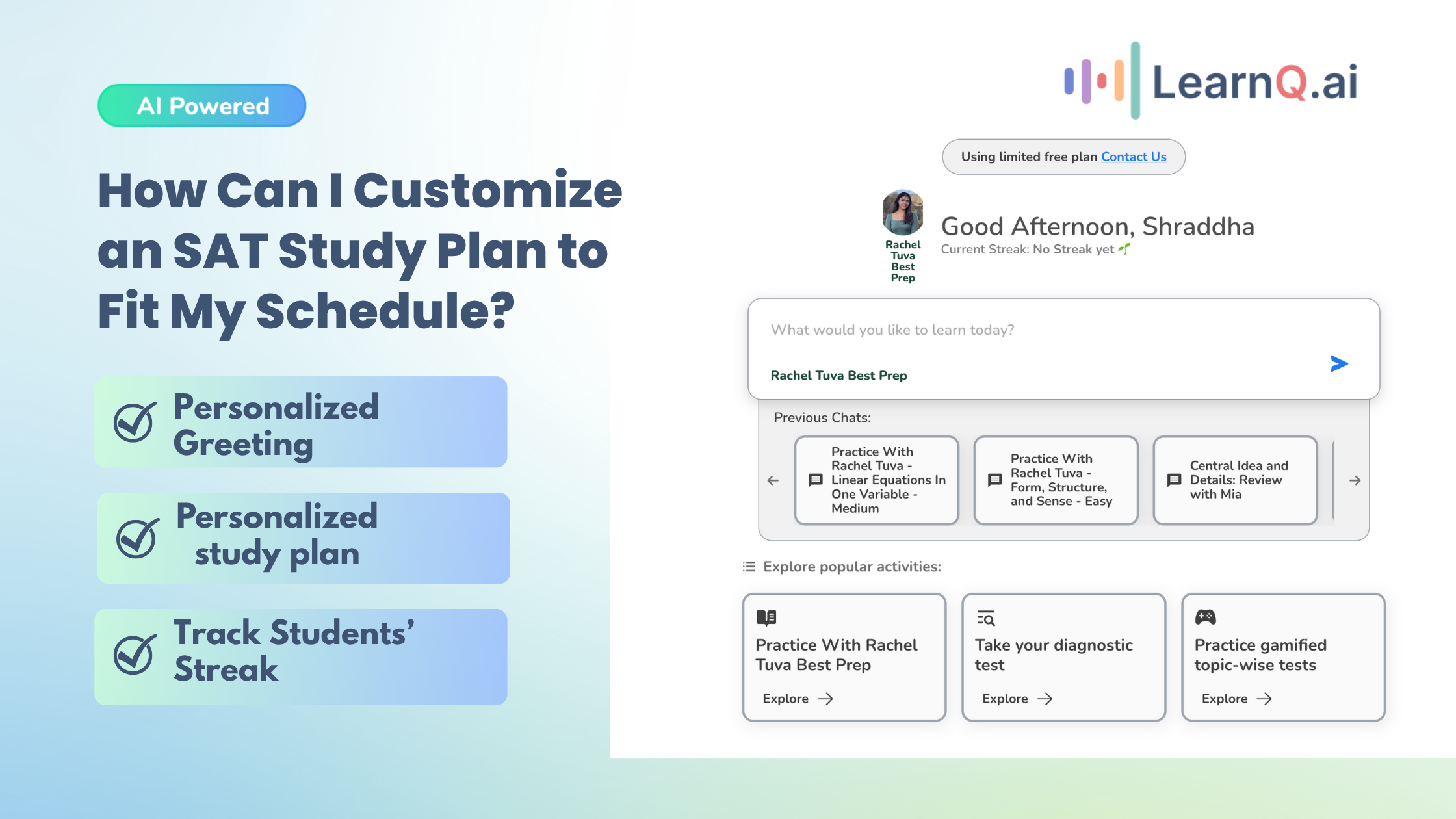
Practice with LearnQ.ai
While this blog post provides a solid foundation of SAT algebra questions, there’s a wealth of resources available to help you master algebra for the SAT and beyond. Consider exploring LearnQ.ai, a game-changing online SAT prep course designed to make learning fun and effective.
Here’s why LearnQ.ai is the perfect partner for your SAT algebra questions conquest:
- Adaptive Learning: LearnQ.ai utilizes an intelligent algorithm that tailors the learning experience to your strengths and weaknesses. It identifies areas where you need more practice and provides targeted instruction to bridge the gaps.
- Gamified Practice: Learning shouldn’t feel like a chore! LearnQ.ai incorporates engaging game mechanics to transform algebra practice into an interactive adventure. You’ll earn points, unlock levels, and compete with friends, all while solidifying your algebra concepts.
- Full-Length Practice Tests: With LearnQ.ai, you’ll have access to a vast library of full-length practice questions designed to mirror the format and difficulty of the actual SAT Math section. The more you practice, the more confident you’ll become on test day.
- Diagnostic Tests: Get a clear picture of your current algebra skills with LearnQ.ai’s diagnostic tests. These targeted assessments pinpoint your strengths and weaknesses, allowing you to focus your studies on the areas that need the most improvement.
- Mia – AI Tutor: LearnQ.ai’s AI tutor, Mia, is always by your side to provide instant help and personalized guidance. Ask Mia questions, get explanations for challenging concepts, and receive feedback on your work. Mia is a tireless companion on your journey to mastering algebra.
Ready to take your algebra skills to the next level?
Sign up for free on LearnQ.ai today and experience the fun and effective way to conquer the SAT Math section!